If the next illustration, from an Indianapolis Children's Museum exhibit, is correct, then the driver's strategy might work.


If the next illustration, from an Indianapolis Children's Museum exhibit, is correct, then the driver's strategy might work.

Unfortunately,the above illustration violates the law of conservation of momentum. Merging flows at the rear should maintain downward momentum. Also the principle of action-reaction, which would require upward lift to be equaled by downward accelerative force on the air, is not evidenced in departing air which has no net deflection.
Plausible falsehood is still being taught. The most popular theory of wing operation, which we may call Hump Theory, because it requires a wing to have a more convex upper surface as compared to the lower, is easily shown to be false. Hump theory is based on Bernoulli's law, according to which pressure and velocity are inversely related, and on a principle of equal transit times, according to which air passage over an upper wing surface must occur in the same time as air passage below. In order to have the same transit time, flow at a more curved upper wing surface, having a longer path, is said to be of greater velocity than that at a less curved lower surface, making upper surface pressure less than that at the lower, in accordance with Bernoulli's law.
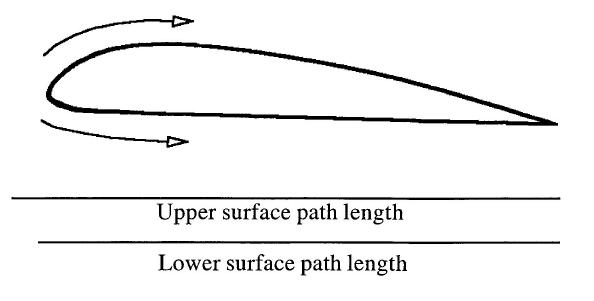
Upper surface flow is indeed faster than the lower, so much so that transit time at the upper surface in typical normal flight is always LESS than at the lower. Although Bernoulli's law is sound and well proven, the premise of equal transit time is invalid and without foundation in known physics. Thus the most popular explanation, world-wide, of wing operation is false, and easily shown to be so.
Hump theory does not allow for balsa toy gliders with flat wings, which have equal upper and lower surface path lengths. Lift is, without question, due to pressure being greater at the lower surface than at the upper surface. Thus according to Bernoulli's law, air transit time at the upper surface must be less than at the lower. This refutes the hump theory argument that upper and lower transit times must be equal. Hump theory also does not allow for inverted flight of aerobatic airplanes, which have equal upper and lower wing surface curvatures.
For mathematical test of hump theory, consider one the most popular trainers, the Cessna 150 or 152, which has wings of 160 square feet total area, upper surface path about 1.6 percent longer than the lower, and can fly, flaps-up, at 55 miles per hour, or 81 feet per second. We can calculate lift according to hump theory. Let's assume near sea level air density of about .0024 slug per cubic foot, and assume no change in below-wing pressure or flow velocity, as hump theory explanations usually do. If upper surface velocity is increased by 1.6 percent in order to attain equal transit time, the change is .016 times 81, or 1.296 feet per second, making upper surface velocity 82.296 feet per second.
Now let's plug that information into the Bernoulli expression which says pressure difference is equal to one half density, times difference between initial velocity (airplane airspeed) squared and upper surface velocity squared. Pressure difference between upper and lower surfaces then is (1/2)x.0024 x [(81 squared)-(82.296 squared)]. The upper surface pressure reduction then becomes .2544 pounds per square foot. Multiplying this by wing area of 160 square feet gives total lift of 40.7 pounds, a small fraction of the 1600 pounds rated gross weight of the airplane. Minimum flaps-up flying speed for the airplane according to hump theory would be over 300 miles per hour, well above the redlined dive speed of 160 miles per hour.
If air velocity were increased because of a wing upper surface hump, it would be reasonable to expect above-wing velocity increase only directly above the wing. However, as shown in the following figures, velocity is affected even ahead of a wing.
Relative velocities were measured above and below wing level in increments of one foot, and at distance one-half foot ahead of the leading edge. In the plot, the wing leading edge position represents airplane airspeed. Pitot tube airspeed plotted forward of leading edge position indicates relative velocity decrease due to forward movement of air as the wing passes. Pitot tube airspeed data greater than airplane airspeed, as plotted behind leading edge position, indicates rearward movement of air as the plane passes. Behind the plane air has followed the wing contours to depart in downward direction.
All air movement is circulatory. Ahead of the wing, air drawn toward lower above-wing pressure pressure and away from greater below-wing pressure rises ahead of the wing. In total then, upward movement ahead, rearward movement above, forward movement below and downward movement behind constitute a circulatory movement traveling with the wing, which is known as "circulation."superimposed on passing flow.
Aerodynamics teaching at college level, where lift calculations must correspond with the real world, disregards hump theory because it is clearly false, and instead is based on circulation theory. We can reasonably assume that many who have studied aerodynamics in college were previously taught hump theory in lower level schooling and now know it is wrong. We may justifiably wonder why some of these better educated persons have not returned to enlighten the lower levels with more appropriate teachings? Perhaps it is because higher level teaching also has questionable aspects.
Although normally presented in highly mathematical context, classical basic wing theory at the college level can be expressed in simple terms without math. According to classical theory, a wing begins to produce lift when a "starting vortex" is left behind as the wing moves forward. This vortex is said to, by a somewhat vague process, cause "circulation" to appear around the wing.

With circulation superimposed on passing flow so that upper surface air flow velocity is greater than that below, lift can be explained, as in hump theory, in connection with Bernoulli's law. A flaw in this explanation is that physical principles explaining how the starting vortex causes wing circulation is a bit hazy. Other invocations of induction without sound physical reasoning produce classical terms of "induced downwash," "induced angle of attack," and "induced drag." This classical explanation is a sort of mathematical analogue, adopted from electromagnetic theory. It doesn't really explain the physics, but is nevertheless quite useful in that the equations from electromagnetism produce results corresponding to the real world of wing operation.
Unfortunately, or fortunately, depending on point of view, hump theory provides a "plausible" explanation of lift for the non-mathematical student, while the mathematics of electromagnetic theory and electromagnetism, from which induction concepts arise, adapts well in wing performance calculations. Thus flight instructors have an explanation for flying students, aerodynamicists and engineers find little reason to question the physics of calculations which seem to work, and physicists interested in more exotic research may have little interest in mundane mechanics of popular aerodynamics, where the starting vortex does indeed develop, and simultaneously above-wing flow develops greater velocity. These phenomena, however, are easily accounted for without invoking theinduction effects of electromagnetism.
Aerodynamic lift of a wing can be explained and calculated through simple application of Newtonian physics. Air flow following the contours of a wing in normal flight departs in a downward direction. In this redirection of flow, downward momentum is produced. Upward reaction force (or lift) must be equal, according to Newtonian physics, to the downward rate of change of air momentum. Inclination of a wing lower wing surface deflects some air downward there, while greater downward deflection is produced as flow follows the downwardly-curving upper surface. In the downwardly-curving flow, an upward pressure gradient exists which opposes atmospheric pressure to cause upper surface pressure reduction. Bernoulli's law is satisfied with velocity changes related to pressure changes when oncoming air accelerates over the wing leading edge into the reduced pressure above the wing and decelerates in encounter with increased pressure below the leading edge. The pressure difference also accelerates air upward around the leading edge. These accelerations occur in accordance with Bernoulli's law, but the greater upper surface velocity is more easily explained as resulting from pressure difference, rather than causing it as popular theories teach.
As air is accelerated downward by wing passage, upward recirculation occurs all around the airplane, away from higher pressure below and toward lower pressure above. Thus recirculation occurs forward and upward around the wing, and laterally outward,upward and inward to produce twin trailing vortices which is made visible in smoke behind aerobatic plane wings at airshows.
Forward recirculation carries upwash into which the wing flies. Near stall condition, upwash velocity rounding a wing leading edge can be greater than the forward velocity of the airplane, pushing a stall warning switch tab, as shown here on a Cessna 182, forward and upward to operate a stall warning horn.

Energy is recovered from leading edge upwash as circulation rounds the leading edge to produce centrifugal pressure reduction, known as "leading edge suction," and forward thrust. Leading edge suction is sometimes used to operate a stall warning horn, as on this Cessna 175.

Leading edge pressure reduction produces forward thrust on the wing, but curvature of circulation around the rear produces opposing rearward thrust. If these were equal they would cancel, but energy lost into lateral recirculation around the wing ends causes forward thrust to be less than rearward thrust. The difference between these thrusts appears as drag, commonly referred to as "induced drag," because the classical mathematics treatment is similar to that of electromagnetism and electromagnetic induction.
>
A 1999 book on the subject, titled "Stop Abusing Bernoulli!-- How Airplanes Really Fly," ISBN 0-9646806-2-9. Was written by this author.
 Advertised in Sport Aviation Magazine, the book was sold to many countries of the world and was translated into Korean for use in Pusan National University.
Advertised in Sport Aviation Magazine, the book was sold to many countries of the world and was translated into Korean for use in Pusan National University.

That book has been superseded by a second book with more detailed presentation, published in 2003, titled Introduction to Aerodynamics ISBN 09646806-3-7. In hardcover with 224 pages and 167 illustrations, it can be ordered from your local book store, the Academy of Model Aeronautics museum book store in Muncie, Indiana, phone 765-289-4236, or from Amazon.com. Sample pages can be found on Amazon.com by clicking here.
 Author Gale Craig, retired from General Motors Research and Development, holds a Master's degree in physics and is named as sole inventor in sixteen US patents in widely varying areas. He is a pilot of 1600+ hours and owns a Cessna 182.
Author Gale Craig, retired from General Motors Research and Development, holds a Master's degree in physics and is named as sole inventor in sixteen US patents in widely varying areas. He is a pilot of 1600+ hours and owns a Cessna 182.
Comments or questions? galemcraig@aol.com
Copyright 2000 Gale M. Craig
